he interval
![$\left[ A,B\right] $](graphics/notesCF__2__1021.gif) was inserted into the problem (
Example Black
problem
) to avoid considering a PDE problem on the entire real line. We
constructed a basis of functions with finite support, unsuitable for finite
element calculation on unbounded set. We would like to place the numbers
was inserted into the problem (
Example Black
problem
) to avoid considering a PDE problem on the entire real line. We
constructed a basis of functions with finite support, unsuitable for finite
element calculation on unbounded set. We would like to place the numbers
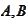 to positions according to the
rule
to positions according to the
rule
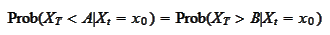 for some number
for some number
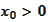 .
The process
.
The process
 is given by the
SDE
is given by the
SDE
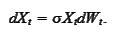 We
calculate
We
calculate
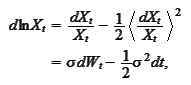
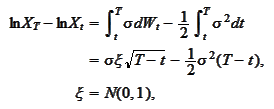
 The distribution density
The distribution density
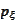 of
of
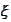 is a symmetric function
is a symmetric function
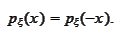 Thus, we take
Thus, we take
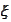 as a controlling
parameter:
as a controlling
parameter:
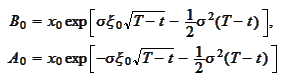 for a fixed positive number
for a fixed positive number
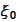 .
.
The only remaining step is to make sure that the interval
![$\left[ A,B\right] $](graphics/notesCF__2__1036.gif) is adapted to binary structure of wavelet basis. We choose a scale parameter
is adapted to binary structure of wavelet basis. We choose a scale parameter
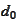 then
then
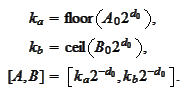
The parameter
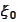 should be taken so
that
should be taken so
that
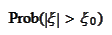 would be comparable to precision of initial approximation of the payoff
function, discussed in the section
(Decomposition
of payoff function in one dimension
). The parameter
would be comparable to precision of initial approximation of the payoff
function, discussed in the section
(Decomposition
of payoff function in one dimension
). The parameter
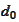 should be taken to be the minimal number needed to provide the condition
(
Sufficiently fine scale 2
).
should be taken to be the minimal number needed to provide the condition
(
Sufficiently fine scale 2
).
|