et
 be a probability space. It is natural, after results
(
Incomplete market ask
) and
(
Incomplete market bid
), to consider a
mapping
be a probability space. It is natural, after results
(
Incomplete market ask
) and
(
Incomplete market bid
), to consider a
mapping
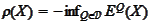 where the
where the
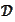 is some set of absolutely continuous with respect to
is some set of absolutely continuous with respect to
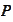 measures.
measures.
Proposition
The coherent measure has the following properties:
Proposition
Proposition
1. (Subadditivity)
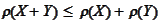 .
.
2. (Monotonicity)
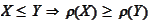 .
.
3. (Positive homogeneity)
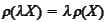 for
for
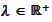 .
.
4. (Translation invariance).
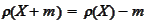 .
.
5. (Fatou property).
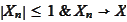 in
in
 .
.
Note that if
 is finite then the representation (
Coherent
measure
) is exactly the formula (
Support
function
). Hence, for the finite
is finite then the representation (
Coherent
measure
) is exactly the formula (
Support
function
). Hence, for the finite
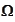 the representation (
Coherent measure
)
completely describes all functions that have the property 1 and 3.
Furthermore, if we introduce a notion of maximal set
the representation (
Coherent measure
)
completely describes all functions that have the property 1 and 3.
Furthermore, if we introduce a notion of maximal set
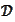 (in inclusion sense) for a measure
(in inclusion sense) for a measure
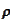 then, given
then, given
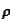 and
and
 as in (
Coherent measure
), taking double
convex dual of
as in (
Coherent measure
), taking double
convex dual of
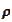 reveals that the
reveals that the
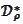 is a closure of the convex hull of the original
is a closure of the convex hull of the original
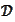 .
Another description for
.
Another description for
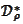 is
is
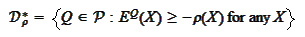 where the
where the
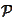 is the set of all absolutely continuous measures with respect to
is the set of all absolutely continuous measures with respect to
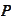 .
.
In the case of general
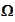 there is a following representation theorem.
there is a following representation theorem.
Proposition
A measure
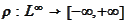 satisfies the conditions 1-5 above iff there exists a non empty
satisfies the conditions 1-5 above iff there exists a non empty
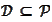 such that the (
Coherent measure
) holds.
such that the (
Coherent measure
) holds.
Note that the theorem holds for
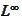 .
There reason may be easily understood by looking at derivations around the
formula (
Support function
). To extend those
derivations to the general case we need the duality to the space
.
There reason may be easily understood by looking at derivations around the
formula (
Support function
). To extend those
derivations to the general case we need the duality to the space
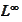 in order to make sense of all the scalar products
in order to make sense of all the scalar products
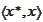 .
Such duality needs to have reflexivity. The
.
Such duality needs to have reflexivity. The
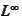 has such dual space
has such dual space
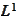 while
while
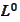 ,
endowed with the topology of convergence in probability, does not have such
structure.
,
endowed with the topology of convergence in probability, does not have such
structure.
|